The unique Dutch pandemic strategy of "maximum control" (controlled spread) emerges from the methods used by RIVM (Dutch CDC) to model the course and interventions of COVID-19 assume immunity-building. There is no room for a containment strategy with test-trace-isolate, while many countries have had demonstrable success with a containment strategy. Also, effective immunity building is out of the question. The article is technical and does require a bit of concentration, but the summary is written in plain English, also for laypersons.
As a chemical engineer in his own field, Jorrit Posthuma de Boer is very familiar with this type of modeling, and explains in this article how the RIVM models work and what weaknesses they have. This article is based on this PowerPoint and this PowerPoint, which were part of the presentations of RIVM (Dutch CDC) Director Prof. Jaap van Dissel on March 18th and March 25th in Parliament.
Summary (also for the mathematically disinclined)
As you are aware, governments use calculation models to determine the course of action. After thorough analysis, we have been able to recreate the basic structure of the models the RIVM uses. It turns out many of the ideosyncracies and imperfections of the model have informed Dutch policy. And so we now know how the model has informed (or forced) the many unfortunate decisions and statements by the Dutch authorities:
- The RIVM could not model China's fast containment as "hard work in testing and isolation", so assumed it was quick Herd Immunity. It was assumed there is an "iceberg" of people who had the disease, are immune, but didn't notice.
- Test-trace-isolate as a containment technique is difficult to model and Containment comes out as a full year of lockdown, hence the choice to focus on immunity buildup towards Herd Immunity
- The statements about children not being susceptible or transmission vectors led to decisions about opening schools
- The view on transmission as through droplets only informed social distancing rules
- The model pulls thinking in the direction of ignoring the significant suffering and health damage outside of hospitals.
It now seems that the Netherlands embarked on the immunity buildup through controlled exposure experiment because a flawed model convinced the modeler to advise a course of action, which was accepted wholesale by the RIVM, whose advice was taken over by the Outbreak Management Team advisory council, whose advice was taken over by the Government, which has somehow been able to get the strategy past the media and public for three months now.
An interesting model
During the briefing to the House of Representatives, Jaap van Dissel, director of the Center for Infectious Disease Control at RIVM, presented three scenarios for tackling the corona virus in slide 7 of his PowerPoint presentation, based on the modeling we will analyze in this article.
The results are somewhat strange: the tail of the 'no interventions' scenario (the red line) descends much steeper than that of the 'lock-down' and 'maximum control' scenarios. This is notably strange.
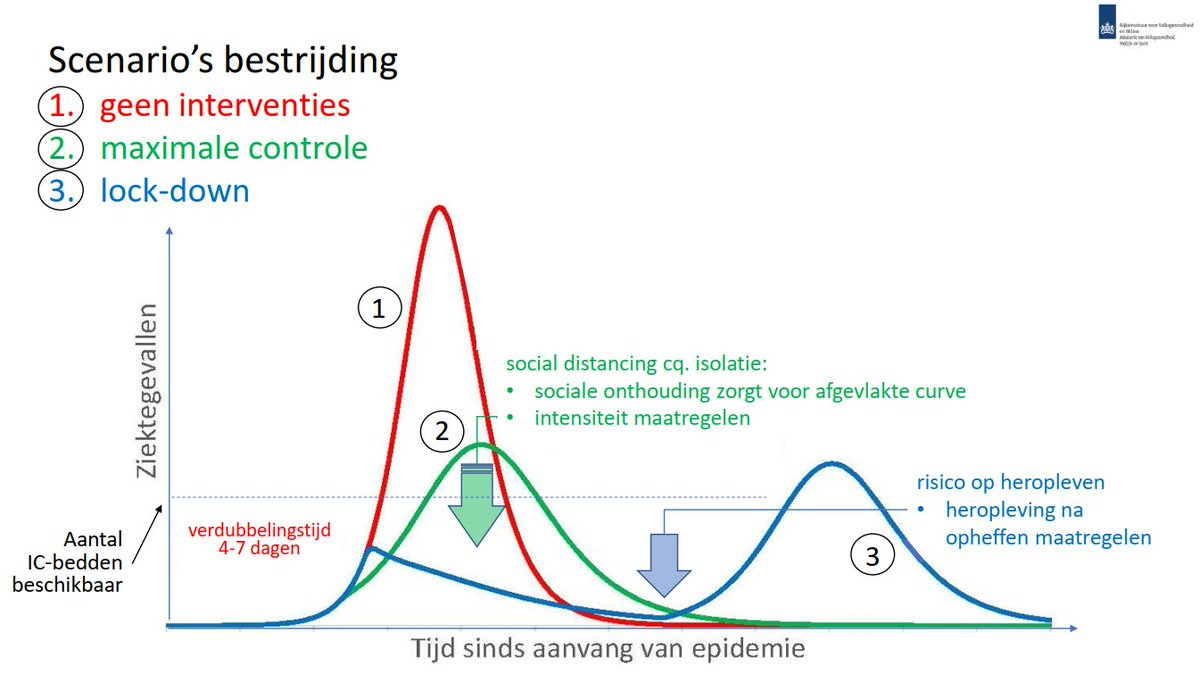
Chart legend: Bestrijding = outbreak control; geen interventies = no interventions; ziektegevallen = number of afflicted people; aantal IC-bedden beschikbaar = number of ICU beds available; verdubbelingstijd = doubling period; sociale onthouding zorgt voor afgevlakte curve = social distancing leads to flattened curve; intensiteit maatregelen = intensity of measures; risico op heropleven = risk of resurgence; heropleving na opheffen maatregelen = resurgence after lifting of measures; tijd sinds aanvang epidemie = time elapsed since start of epidemic.
To investigate what is going on, we must explain the SEIR model which is often used for epidemiological modeling. Its components are:
- S for the number of persons susceptible to the disease ("Susceptible"), i.e., the number of persons who are not yet immune to the disease;
- E for the number of people who have become infected at any time ("Exposed");
- I for the number of persons who are infectious ("Infected"), i.e., who can transmit the disease to others;
- R for the number of people who are recovered and immune to the disease ("Recovered").
Schematically this model looks as follows:
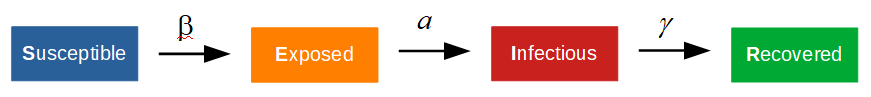
The SEIR model is a simplification. But more importantly, this model does not reflect the consequences that a disease can have. The model does not take into account that there will be persons who will have permanent injury from the disease and will therefore become incapacitated for work and / or need permanent care. This will entail suffering and other social costs, including long-term care.
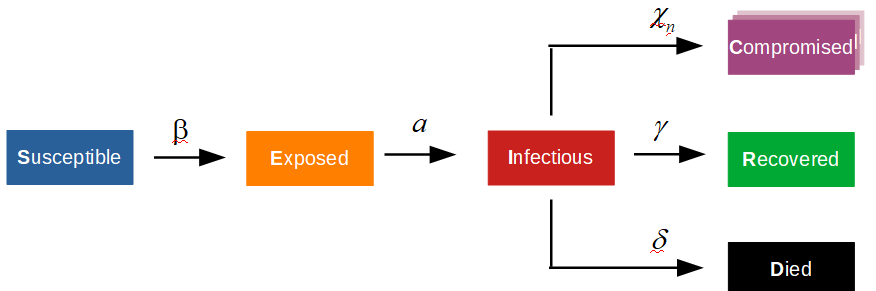
People can also die of an illness. It is important for policymakers that these 'side effects' of a disease are also made transparent. This is possible with the model shown below, which also includes permanent injuries in degrees ('Compromised') and mortality ('Died').
Let's return to Slide 7 (March 18, 2020). It is strange that the 'no interventions' scenario is steeper than the 'lock-down' scenario. Apparently, RIVM assumes that an immunity feedback will occur, as a result of which the disease transmission is strongly inhibited.

Does the RIVM in its modeling assume a parallel transmission route via asymptomatic contamination? This would be presented as the model below:
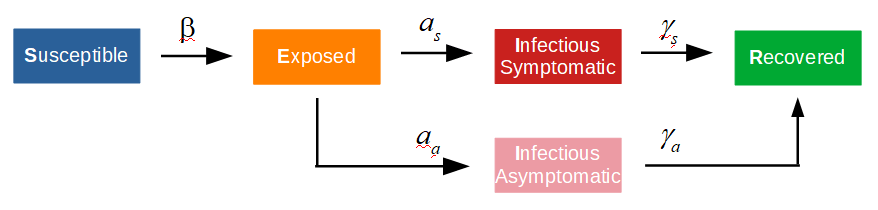
If one assumes that asymptomatic infection proceeds less quickly than symptomatic infection, a group immunity strategy becomes very attractive. This can be seen in slide 3 (March 18), in which the "Infection Pyramid" was presented. For 81% of the infected persons, an 'asymptomatic' clinical picture was expected.
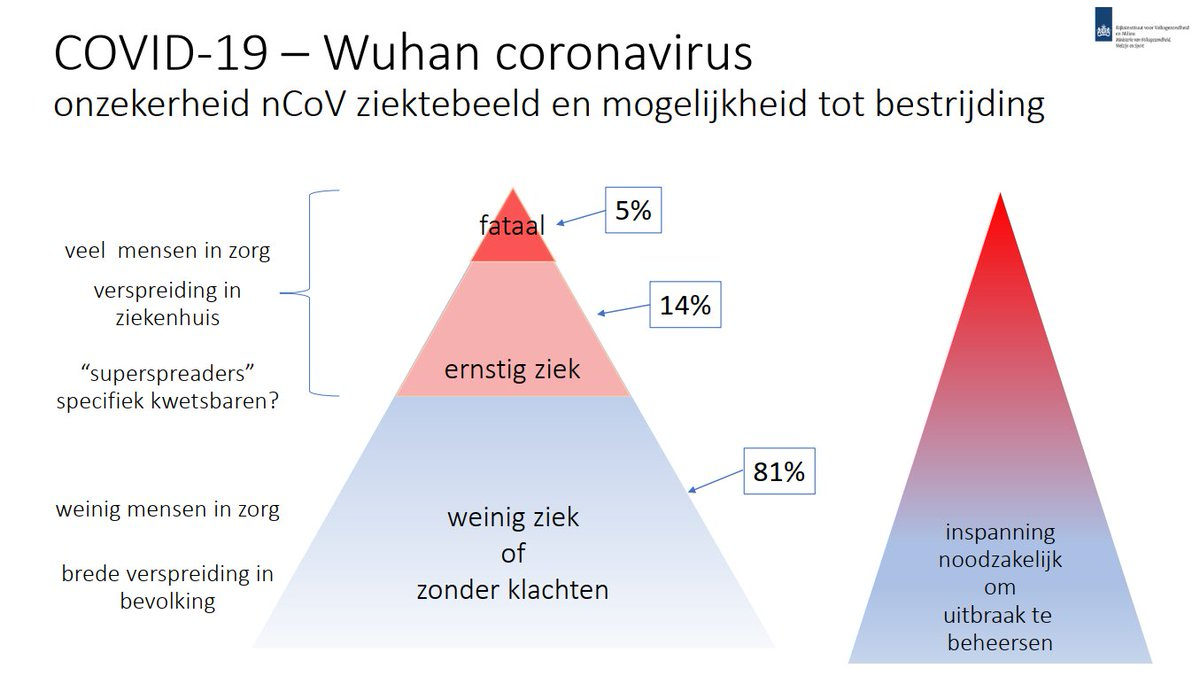
Legend of the Slide: onzekerheid nCoV ziektebeeld en mogelijkheid tot bestrijding = uncertainty about manifestation of the nCoV disease and possibility of control; veel mensen in de zorg = many people in hospital; verspreiding in ziekenhuis = spread in hospitals; specifiek kwetsbaren = specifically vulnerable; weinig mensen in zorg = few in hospital; brede verspreiding in bevolking = broad spreed in population; fataal = fatal; ernstig ziek = seriously ill; weinig ziek of zonder klachten = mild ilness or no symptoms; inspanning noodzakelijk om uitbraak te beheersen = efforts required to control the outbreak.
If it is assumed that only droplet (as opposed to aerosol or contact) infections contribute to disease transmission, the model can be further simplified. This is because 'asymptomatic' contamination no longer contributes. There are now two routes that reduce the number of infected (E).
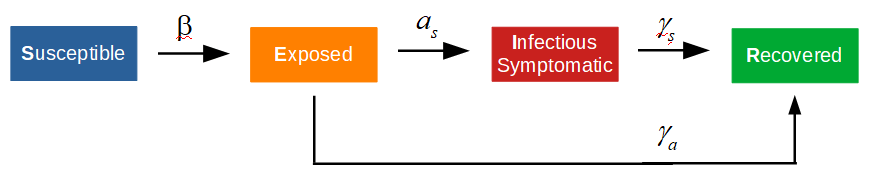
As a result, there will be fewer infectious agents (I) that contribute to the disease transfer, i.e., the disease transfer is inhibited. This especially if the parameter 'a_s' is significantly smaller than 'gamma_a'.
However a TTI scenario looks analogous, because in this scenario people are also removed from the Exposed reservoir and isolated, putting a brake on disease transmission. The TTI model will look like this. 'Infectious (in circulation)' is the number of free-roaming infectious persons and 'Infectious (isolated)' is the number of persons who are both infectious and quarantined.
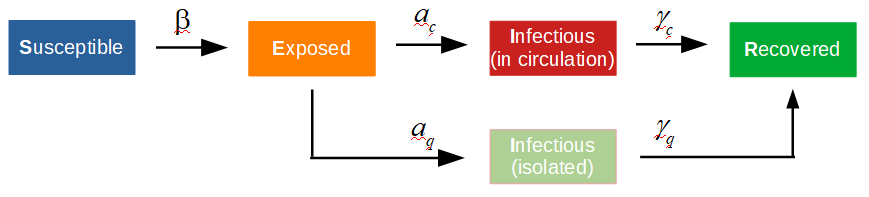
Van Dissel seems to say here that the modeling results from China (Wuhan) could only be explained by building up immunity.
China's curve is as follows:
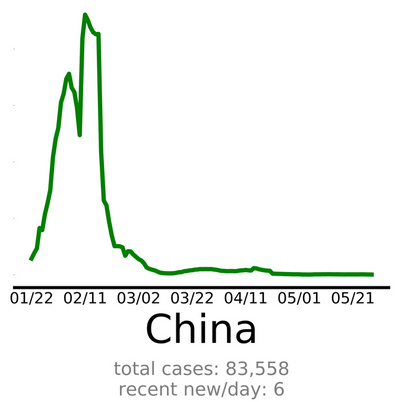
China's peak spans a relatively short period of time. However, the abrupt attenuation, i.e., withdrawal from the 'Exposed' reservoir, was not caused here by immunity building, but by a solid 'track-trace isolate' policy. For comparison, the NL curve:
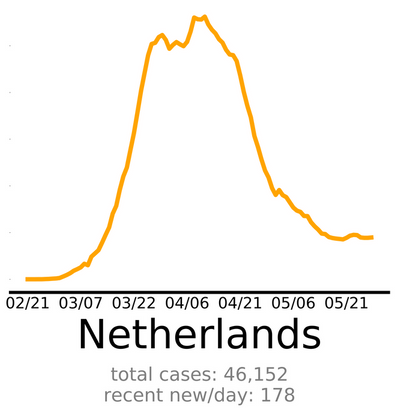
The Dutch peak is significantly wider than the peak in China. In this sense, the Chinese have been more successful in keeping the 'Exposed' reservoir small. The damping in the Netherlands by building up immunity will have been marginal, since antibodies have been 'demonstrated' by Sanquin in only 5.5% of the Dutch population. Also, immunity has not yet been proven and any immunity will probably last only a few months. If (rapid) immunity build-up contributes to the inhibition of disease transmission, this should be visible in the curve of Sweden:
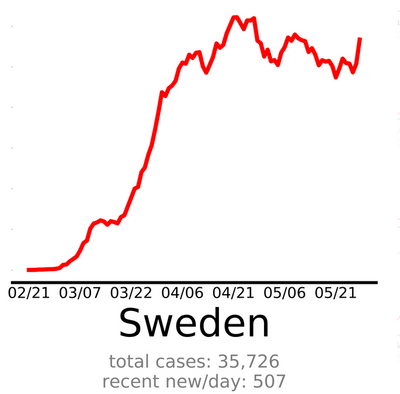
So far, the curve of Sweden has not provided any indication of a rapid transfer inhibition. My preliminary conclusion is that in its modeling presented on March 18, RIVM has assumed a model that is analogous to the model reproduced below:

Infectious Symptomatic = Infectious (Schedule - Red Block)
The presented scenario (Slide 7, technical briefing of March 18) comes from the following publication: How will country-based mitigation measures influence the course of the COVID-19 epidemic?
The model used is shown schematically in the accompanying supplementary material.
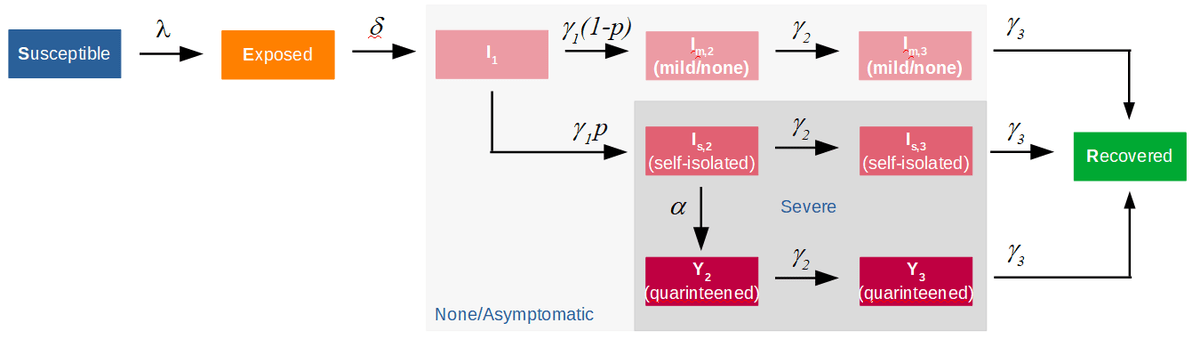
Indeed, the model contains a parallel route via asymptomatic and pauci-symptomatic patients. The model also includes the possibility to simulate self-isolation, based on low effectiveness, and quarantine. However, only for 'seriously' ill patients.
In short, isolation/quarantine of asymptomatic and pauci-symptomatic patients is not possible in this model. The article describes that at the time, 80% of asymptomatic and pauci-symptomatic patients were assumed, which cannot be isolated in this model. A track-trace isolate policy cannot be simulated with the model shown in the supplement.
If this model is fitted to the data possibly available from Wuhan or Shenzen, this will lead to parameters that will exaggerate the asymptomatic and pauci-symptomatic pathway.
Age cohorts
The newspaper het NRC (22-3-2020) contains a more detailed description of the models used by RIVM. Age cohorts are used. This means that for each age cohort an epidemiological model is drawn up with parameters specific to that age group. Most of these parameters can be determined/estimated from published or exchanged data.
A required hospitalization can be used as a definition for a seriously ill patient. From the available data regarding hospital admissions (shown below for IC), the share of severely ill patients can be determined / estimated per age cohort.
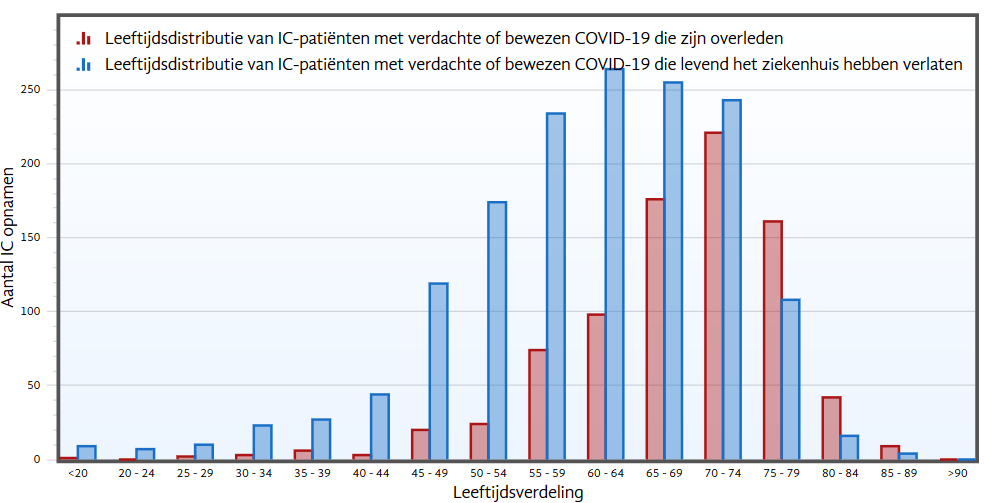
Legend of the chart: aantal IC-opnamen = number of ICU admissions; Leeftijdsdistributie = age distribution; verdachte of bewezen = suspected or confirmed; overleden = dead; levend het ziekenhuis hebben verlaten = have left the hospital alive.
Roughly speaking, it follows from the above figure that the clinical picture appears to be not too bad for the younger age cohorts, but this is not the case for the older age cohorts. It should also be noted that the long-term effects are not clear at the moment. Nor does this seem to have been included in policy-making.
With only a bit of exaggeration, this means that the following epidemiological model describes the younger age cohorts:
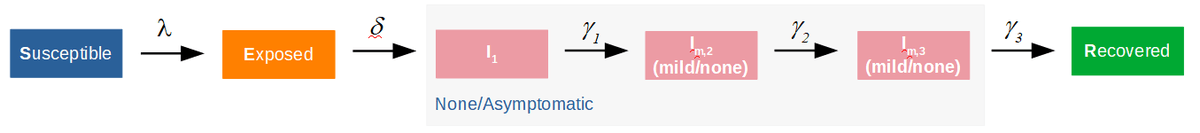
For the younger cohorts, no track-trace and isolation (TTI) is possible in the epidimiological model. This is because it is assumed that the clinical picture for these cohorts is mild. However, the model does not have a TTI option for this disease (original source of the graphic.
Hope of immunity buildup?
The op-ed article "Test for antibodies, that is now essential" by epidemiology researcher Jaap Goudsmit in the NRC newspaper shows that around 7 April 2020 there was great interest in the extent to which the NL population had produced antibodies. The economy also seems to prevail over public health: "The sooner social interaction gets going again, the better", at least that was the feeling among the experts. The serological tests were therefore ordered in no time.
The serological test results were very disappointing, only 5.5% +/- 0.5% in May (3% in April). Alternative immunity markers are now being looked for, e.g., pre-existing SARS-CoV-2 cross-reactive T-cells and the like.
The problem is that it is now completely unclear how many Dutch people have built up immunity so far. Policy makers and experts may still hope that a significant part of the population has built up immunity. That is why we are now slowly lifting interventions to experiment to see whether that immunity has actually been built up. With all the risks that entails.
Supplement RIVM COVID-19 modeling:
RIVM actually uses a system of ordinary differential equations (belonging to the SEIR model) for each age cohort. They probably use different parameters for each age cohort. This produces even more parallel routes.
The residence time can thus be regulated in each container and per age cohort. It can be assumed that the younger cohorts have a shorter duration of illness. This creates, as it were, an additional parallel flow through the younger age cohorts. RIVM probably had to do this to be able to simulate the sharp Wuhan.
Track-trace isolate (TTI) was used in China. This is mathematically somewhat difficult to describe. In the beginning there are start-up problems (here in NL already 6 months), but when the peak is over, TTI becomes increasingly efficient. There are also fewer and fewer infected people. This makes the decrease not exponential but much faster. RIVM could not describe that well with the influenza model.
This may also be the explanation that the figures from China were not believed.